항공기 구름 관측에 사용되는 전방산란 관측 기기의 정확도 향상을 위한 구름입자의 광학적 특성 계산

1. 서 론

구름이 지구 에너지 수지에 미치는 영향과 수치 모델에서의 구름의 정확한 표현은 중요하지만 아직 해결되지 않은 문제이다. 구름은 열역학 상(phase)에 따라 액체상(liquid phase), 혼합상(mixed phase), 얼음상(ice phase) 구름으로 구분한다. 구형의 액체상 구름입자(cloud droplet)로 구성된 액체상 구름(물구름)과는 다르게 얼음상 구름(빙운)은 다양한 모양(habit)과 크기의 빙정(ice crystal)으로 구성되어 있다(Bailey and Hallett, 2004; Um and McFarquhar, 2011; Um et al., 2015; Lawson et al., 2019). 혼합상 구름(혼합운)은 액체상 구름입자들과 빙정들이 함께 존재하며 액체상 물과 고체상 얼음에 대한 포화수증기압 차이에 따라 빙정과정(베르게론-핀다이젠-베게너 과정)이 활발히 일어난다.

빙정이 포함되어 있는 혼합상 구름과 얼음상 구름의 미세물리 특성(microphysical properties)과 복사 특성(radiative properties)를 계산하는 것은 액체상 구름에 비해 상대적으로 어렵다. 이는 빙정이 다양한 종류의 비구형(non-spherical) 모양과 매우 넓은 크기 분포를 갖기 때문이다. 얼음상 구름은 지구 표면적을 최소 17% 덮으며 이는 열대지역에 대해서는 60%에 육박한다(Sassen et al., 2008). 따라서 원격탐사 및 수치 모형에서 필요한 얼음상 구름의 태양 및 지구 복사에 미치는 영향을 알기 위해서는 빙정의 미세물리/복사 특성을 정확히 계산하는 것이 매우 중요하다.

얼음상 구름은 상대적으로 높은 고도에서 존재하기 때문에 직접(in-situ) 관측에는 항공기가 주로 사용된다. 얼음상 구름의 기본적인 특성을 이해하기 위한 가장 기본이 되는 관측은 크기 분포(size distribution) 관측이다. Figure 1은 Tropical Warm Pool-International Cloud Experiment (TWP-ICE) 캠페인 동안 Proteus 항공기에 장착된 cloud droplet probe(CDP, 붉은색)와 cloud imaging probe(CIP, 파란색)로 관측한 얼음상 구름 안에 존재하는 빙정의 크기 분포이다. 일반적으로 빙정의 크기 50 μm를 기준으로 큰 빙정들은 광학배열 관측 기기(optical array probe, OAP)로 관측을 수행하고 작은 빙정들은 전방산란 관측 기기(forward scattering probe)를 사용하여 수농도(number concentration), 크기, 또는 모양을 관측한다. 광학배열 관측 기기는 연속된 복수의 다이오드(diode) 배열 위로 구름입자가 지나가면 레이저 빔을 가리게 되고 다이오드에 구름입자의 그림자가 생기게 된다. 이 그림자의 크기와 모양을 연속적으로 저장하여 구름입자의 크기, 모양, 수농도를 결정한다(Wendisch and Brenguier, 2013; Baumgardner et al., 2017; McFarquhar et al., 2017). 초기에 널리 사용했던 광학배열 관측 기기는 Particle Measuring Systems (PMS)사의 two-dimensional cloud (2D-C, Knollenberg 1970, 1976, 1981) spectrometer와 two-dimensional precipitation (2D-P, Knollenberg 1970, 1976, 1981) spectrometer가 있으나 더이상 생산하지 않는다. 현재 전세계적으로 많이 사용하는 광학배열 관측 기기는 Droplet Measurement Technologies (DMT, www.droplet-measurement.com)사에서 개발한 CIP (Baumgardner et al., 2001, Fig. 2)와 precipitation imaging probe (PIP, Baumgardner et al., 2001)가 있다. 대한민국 기상청 King Air 항공기에는 CIP와 PIP가 장착되어 있다(Cha et al., 2019). Stratton Park Engineering Company (SPEC, www.specinc.com)사에서 개발한 two-dimensional stereo spectrometer (2D-S, Lawson et al., 2006)와 high volume precipitation spectrometer (HVPS, Lawson et al., 1998) 또한 많이 사용하고 있는 광학배열 관측 기기이다. 일반적으로 광학배열 관측 기기는 10~50 μm의 해상도를 갖는다.

Fig. 1.

An example 30 sec averaged particle size distribution measured by a cloud droplet probe (CDP, red) and a cloud imaging probe (CIP, blue) during the 2006 Tropical Warm Pool International Cloud Experiment (TWP-ICE). The measurement was made at 065730 [HHMMSS] UTC on January 29, 2006 with an average temperature of -48.63oC.

Fig. 2.

Pictures of a cloud imaging probe (CIP, left) and a cloud droplet probe (CDP, right). The CIP laser is illuminating on a finger near the sample area of CIP. An air spray with a calibration tool kit (black plastic plate) are also shown with the CDP.

구름입자가 전방산란 관측 기기의 관측 영역(sample area)를 지날 때 레이저 빔에 의해 산란 현상이 일어난다. 이때 특정 각도로 산란된 빛의 강도를 기반으로 구름입자의 크기를 역산출 할 수 있다(Wendisch and Brenguier, 2013; Baumgardner et al., 2017; McFarquhar et al., 2017). 이는 입사하는 빛의 파장(λ)과 산란체(scatterer)의 모양과 굴절률(refractive index)을 아는 경우, 특정 산란 각도로 산란된 빛의 강도로부터 산란체의 크기를 산출할 수 있다는 이론을 기반으로 한다. 전방산란 관측 기기의 최초 모델은 PMS 사에서 개발한 forward scattering spectrometer probe (FSSP) 100이다(Knollenberg, 1976, 1981). 이후 DMT사의 CDP (Lance et al., 2010, Fig. 2), cloud aerosol spectrometer (CAS, Baumgardner et al., 2001), cloud and aerosol spectrometer with polarization (CAS-POL, Glen and Brooks, 2013), cloud particle spectrometer with polarization detection (CPSPD, Baumgardner et al., 2014), SPEC사의 fast FSSP (FFSSP)와 fast CDP (FCDP) 등이 개발되었다. 기상청 King Air 항공기에는 DMT 사의 CDP가 장착되어 2~50 μm 크기의 구름 입자의 크기와 수농도를 관측한다.

본래 전방산란 관측 기기는 작은 구형의 액체상 구름입자를 관측하기 위해 개발되었으나 현재 빙정 관측에도 사용되고 있다. 전방산란 관측 기기를 사용하여 빙정을 관측 시 매우 큰 문제가 발생한다. 빙정은 고체상의 물이라 액체상 물과는 굴절률이 다르며 또한 다양한 비구형 모양과 크기를 갖기 때문에 액체상 구름입자 관측에 사용된 미 이론(Mie theory)에서 가정한 구형의 액체상 구름입자와는 광학적 특성이 매우 다르다. Borrmann et al. (2000)과 Meyer (2012)는 T-matrix (Mishchenko et al., 1996; Mishchenko and Travis, 1998) 방법을 사용하여 원기둥(cylinder)과 회전타원체(spheroid) 모양을 가정한 빙정의 단일산란 특성(single-scattering properties)을 계산했다. 이 계산을 통해 비구형 빙정을 전방산란 관측 기기로 관측 시 빙정 크기 산출 오차가 최대 20%에 달한다는 것을 보고하였다. 이 연구들의 문제점은 빙정의 모양을 회전대칭(rotationally symmetric)인 원기둥과 회전타원체로 가정했다는 것이다. 이는 T-matrix 방법이 회전대칭체에 대해서만 단일산란 특성을 계산할 수 있기 때문이다. 하지만 실제 구름 안에 존재하는 빙정의 모양은 원기둥이나 회전타원체 같은 회전대칭체가 아니라 온도와 습도의 따라 매우 복잡하게 변한다(Bailey and Hallett, 2004; Um and McFarquhar, 2007, 2009; Um et al., 2015; Lawson et al., 2019). Schnaiter et al. (2012)은 구름 챔버 실험에서 생성된 빙정의 최소 60%는 육각기둥(hexagonal column) 모양을 갖고 있는 것을 보고하였다. 빙정의 분자들이 육각 격자 구조(hexagonal lattice structure)를 기본으로 갖기 때문에 육각기둥 또는 육각판(hexagonal plate) 모양이 가장 일반적인 작은 빙정의 모양이다. 따라서 본 연구는 실제 빙정 모양을 갖는 빙정 모형을 사용하여 정확한 단일산란 특성을 계산하고 전방산란 관측 기기로 빙정 관측 시 빙정의 비구형성때문에 발생하는 크기 산출 오차를 정량화함이 목적이다.

2. 전방산란 관측 기기의 작동 원리 및 문제점

2.1 전방산란 관측 기기의 작동 원리

구름입자가 전방산란 관측 기기의 관측 영역을 지나는 순간 구름입자에 입사하는 레이저 빔에 의해 산란 현상이 일어난다. 이때 특정 산란각(scattering angle)에서 산란된 빛의 강도를 측정한다. 대부분의 전방산란 관측 기기는 4~12^o의 산란각을 사용한다(Table 1, Fig. 3). CAS, CAS-POL, CPSPD는 전방산란과 동시에 후방산란(168~176^o)도 함께 관측한다. 여기서 전방산란 관측 기기가 측정하는 빛의 강도는 미분산란 단면(differential scattering-cross section)으로 표현되며 다음과 같이 정의한다.

$\[ C_{sca, \theta }=\frac{1}{k^2}\underset{0}{\overset{2\pi }{\int }}\underset{{\theta }_1}{\overset{{\theta }_2}{\int }}{P}_{11}\sin \left(\theta \right)d\theta d\varphi \]$

(1)

Table 1.

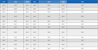
Specifications of forward scattering probes. Determination of typical sample area depends on particle qualification criteria.

Fig. 3.

Scattering phase function P11 of spherical particles as a function of scattering angle calculated using Mie theory. Particle sizes (diameters, Dmax) of 2 μm (left) and 10 μm (right) are shown. Blue and red lines indicate assumed spherical liquid and ice particles, respectively. The light collection angles for forward (4~12o) and backward (168~176o) regions are indicated with gray shadings in each panel.

이때, P₁₁, k, θ, ϕ는 각각 산란위상함수(scattering-phase function), 파수(wavenumber), 산란각, 방위각(azimuth angle)를 의미한다. CAS의 경우 θ₁ = 4^o/168^o, θ₂ = 12^o/176^o이고 CDP는 θ₁ = 4^o, θ₂ = 12^o이다. 미분산란 단면은 산란체의 특성, 크기, 모양에 따라 달라지는 산란위상함수(Fig. 3)와 상대적 관측 위치(즉, θ₁와 θ₂)를 알면 계산할 수 있다. 상대적 관측 위치는 전방산란 관측 기기의 고유한 특성이다(Table 1). 전방산란 관측 기기는 대략 2~50 μm 크기의 구름 입자를 관측하며, 사용하는 레이저의 파장은 액체상과 고체상 물이 비흡수 성질을 보이는 가시광선 영역이다(Table 1). 구형의 구름입자를 가정하였을 때 입사하는 빛의 파장과 반지름 r인 산란체의 상대적 크기 비를 나타내는 크기모수(size parameter, χ = 2πr/λ)는 약 10~240에 해당한다. 이는 미 산란(Mie scattering)과 기하광학(geometric optics) 영역에 해당하며 미 이론을 사용하여 산란체의 단일산란 특성을 계산할 수 있다. 따라서 미 이론을 사용하여 2~50 μm 크기의 구름입자의 미분산란 단면을 계산하면 관측된 미분산란 단면으로부터 구름입자의 크기를 계산할 수 있다. Figure 4는 미 이론을 사용하여 계산한 구형 액체상(Mie liquid, 파란색)과 고체상(Mie ice, 붉은색) 구름 입자의 전방산란 단면(forward scattering-cross section)과 후방산란 단면(backward scattering-cross section)을 비교하였다.

Fig. 4.

Calculated forward (left, 4~12o) and backward (right, 168~176o) scattering cross sections of spherical particles as a function of particle size using Mie theory. Blue and red lines indicate the assumed spherical liquid and ice particles, respectively.

3. 비구형 빙정 단일 산란 특성 계산

비구형인 실제 빙정 모양을 사용하여 전방산란 관측 기기의 빙정 크기 결정시 발생하는 오차 정량화를 위해서는 비구형 빙정의 단일산란 특성(예, 산란위상함수와 산란 단면)을 계산해야 한다. 단일산란 특성을 계산한다는 것은 맥스웰 방정식(Maxwell equation)의 해를 구하는 것이다. 구형 산란체의 경우 정확하고 빠른 미 이론을 사용하여 구할 수 있으나, 비구형인 경우 맥스웰 방정식을 푸는 것은 매우 복잡하고 어렵다. 이는 주로 비선형인 맥스웰 방정식과 경계 조건의 복잡성 때문이다. 회전대칭 모양(예, 원기둥, 회전타원체, Chebyshev particle 등)을 갖는 산란체의 경우 T-matrix 방법을 사용하여 정확한 해를 구할 수 있다. 회전대칭 모양이 아닌 비구형 산란체의 경우 크기모수가 충분이 큰 경우(> ~100) 기하광학 방법(geometric optics method, GOM)을 근사적으로 사용 가능하며(Macke et al., 1996; Um and McFarquhar, 2011, 2015), 크기모수가 작은 경우에는 정확한 해를 구할 수 있는 discrete dipole approximation (DDA)나 i nvariant i mbedding T-matrix method (IITM, Bi and Yang, 2014)를 사용하여 해를 구할 수 있다. 맥스웰 방정식을 정확히 풀어내는 DDA나 IITM은 근사 방법인 GOM에 비해 비교할 수 없을 만큼 많은 계산 자원과 시간을 필요로 한다. 이런 이유로 회전대칭이 아닌 비구형 산란체의 단일산란 특성 계산은 많이 이루어 지지 않았다.

본 연구에서는 육각형 빙정(hexagonal ice crystals)을 단일산란 특성 계산을 위한 빙정 모형으로 사용했다(Fig. 5). 육각형 빙정 모형은 길이(length, L), 폭(width, W), 종횡비(aspect ratio, AR = L/W)로 표현된다. 총 6개의 종횡비, 0.1, 0.25, 0.5, 1.0, 2.0, 4.0를 사용하여 얼음상 구름 안에 존재하는 육각형 빙정의 종횡비 변화폭을 구현했다(Um et al., 2015). 육각형 빙정의 길이와 폭은 각각 최대 48 μm, 20 μm, 크기모수는 최대 ~274까지 사용하여 전방산란 관측 기기의 관측 범위를 포함했다(Table 2). 정확한 단일산란 특성 계산을 위하여 Amsterdam DDA (ADDA, Yurkin and Hoekstra, 2007, 2011)를 사용했다. DDA 계산에서 산란체는 다이폴(dipole)이라 불리는 성분(element)으로 잘게 쪼개져 표현된다(Fig. 5b). 정확한 계산을 위해서는 더 많은 수의 다이폴로 산란체가 표현돼야 하며, 다이폴의 개수가 늘수록 요구되는 계산 시간과 자원은 기하급수적으로 증가한다. 다이폴은 산란체에 비해 현격히 작아야 하며 그 조건은 kd|m| < A로 표현된다. 여기서 d와 m은 각각 다이폴의 지름과 복소 굴절률(complex refractive index)이며, A는 상수이다. 보통의 계산에서는 1.0 정도의 A 값이 요구되나, 본 연구에서는 보다 정확한 계산을 위하여 모든 계산에 A < 0.5를 사용했다. 모든 계산은 0.55 μm 파장에 대해 수행했으며, 해당 파장에서 빙정의 복소 굴절률은 1.311 + i2.289 × 10^-9이다. 전방산란 관측 기기에서 사용하는 레이저의 파장은 약 0.65 μm이다(Table 1). 이는 본 연구에서 사용한 0.55 μm 파장보다 길다. 두 파장에서 액체상과 고체상 물의 흡수율은 모두 0에 가깝고 해당 굴절률의 실수부(real part)의 차이는 작기 때문에 두 파장에 대한 빙정의 단일산란 특성 차이는 매우 작다. ADDA를 사용한 육각형 빙정의 단일산란 특성 계산에는 Um and McFarquhar (2013)에 따라 불규칙 방향(random orientation)을 가정했다. 비구형 육각형 빙정과의 비교를 위한 모든 구형 입자의 계산은 미이론을 사용하여 계산하였다.

Fig. 5.

(a) An idealized model of hexagonal ice crystal with length (L) and width (W). (b) Example hexagonal ice crystals with aspect ratios (AR = L/W) of 0.5, 1.0, and 4.0.

Table 2.

Dimensional information of hexagonal ice crystals used in this study. Maximum dimension (Dmax) is longer of length (L) or width (W). Size parameters defined by Dmax (χDmax = πDmax/λ) and a radius (rv) of equal volume sphere (χv = 2πrv/λ), where λ is a wavelength.

4. 결 과

Figure 6은 ADDA로 계산한 2 μm(왼쪽)와 10 μm(오른쪽) 크기의 육각형 빙정과 미 이론으로 계산한 같은 크기의 구형 구름입자의 산란위상함수(P₁₁)이다. 크기가 50 μm 보다 작은 육각형 빙정이 실제 구름 속에서 가질 수 있는 종횡비의 범위를 여섯 구간으로 나눠서 계산하였다. 구형 구름입자의 경우 액체상(Mie liquid, 검은색)과 고체상(Mie ice, 갈색)을 구분하여 계산하여 그 효과를 확인하였다. 전방산란 관측 기기의 관측 범위인 전방산란 구간(4~12^o)과 후방산란 구간(168~176^o)은 회색 영역으로 각각 표시하였다. 이 구간에서 구름입자의 열역학적 상(액체 또는 고체), 구름입자의 모양(구형 또는 비구형), 크기 및 종횡비에 따라서 산란위상함수의 변화 폭이 매우 넓은 것을 알 수 있다. 특히 후방산란 구간에서 큰 변화폭이 보이나 산란위상함수의 연직 축이 로그 규모임을 고려하면 전방산란된 빛의 강도가 후방산란된 빛의 강도보다 100배 이상 큰 것을 알 수 있다. 구름입자의 크기가 2 μm에서 10 μm로 커짐에 따라 미 이론으로 계산한 구형 구름입자들의 산란위상함수가 더욱 복잡해진다. 이는 앞에서 언급한 간섭과 공명 효과 때문이다.

Fig. 6.

Scattering phase functions P11 of hexagonal ice crystals with varying ARs and spheres (liquid (Mie liquid) and ice (Mie ice)) with maximum dimension of 2 μm (left) and 10 μm (right). The range of forward (4~12o) and backward (168~176o) light collection angles are shaded in each panel.

Figure 6에서 계산한 산란위상함수와 식(1)을 사용하여 전방산란 관측 기기가 관측하는 미분산란 단면을 계산한다(Fig. 7). 미분산란 단면은 전방산란 또는 후방산란에 관계없이 구름입자의 크기가 증가함에 따라 증가하는 것을 보인다. 구형의 구름 입자(Mie ice 와 Mie liquid, Fig. 7)의 미분산란 단면은 진동하는 특징이 강하게 보이나 비구형 육각형 빙정은 그렇지 않다. 전방산란 단면의 경우 크기 약 10 μm 이상의 큰 입자들에 대해서 구형과 비구형에 따른 차이가 지속적으로 증가한다. Figure 7은 다음과 같이 해석할 수 있다. 예를 들어 전방산란 관측 기기가 관측한 전방산란 단면이 40 μm²인 경우(수직 축) 결정된 구형의 액체상(Mie liquid)과 고체상(Mie ice) 구름입자의 크기는 약 15~18 μm이며(수평 축) 하나의 전방산란 단면에 대해 복수의 크기 결정이 가능하다. 종횡비 0.1, 0.25, 0.5, 1.0, 2.0, 4.0의 육각형 빙정을 가정했을 경우 결정된 크기는 각각 약 20.1, 20.2, 20.5, 21.0, 22.0, 28.0 μm이다. 후방산란의 경우 관측된 후방산란 단면에 대해서 가정한 구름입자(예, 액체상 또는 고체상, 구형 또는 비구형, 종횡비)에 따라서 결정되는 구름입자의 크기의 변화 폭이 전방산란의 경우 보다 매우 넓다. 같은 40 μm²의 후방산란 단면의 경우 구형의 액체상(Mie liquid)과 고체상(Mie ice) 구름입자의 크기는 약 8.5~20.5 μm 사이의 복수의 크기 결정이 가능하다. 종횡비 0.1, 0.25, 0.5, 1.0, 2.0, 4.0의 육각형 빙정의 경우 결정된 크기는 각각 약 18-24, 18.5, 13.0, 8.5, 13.0, 23.0 μm이다.

Fig. 7.

Calculated differential scattering cross sections of hexagonal ice crystals and spheres in forward (4~12o, left) and backward (168~176o, right) directions as a function of particle maximum dimension (Dmax). Color schemes are the same as Fig. 6.

Figures 8, 9은 실제 육각형 빙정의 크기(수평 축)와 이를 전방산란 관측기기에서 관측을 하였을 경우 결정된 크기(수직 축)를 비교 하였다. 수직 축의 전방산란 관측 기기에서 관측한 구름입자 크기는 구형 액체상 구름입자를 가정한 미 이론 계산 결과이다. 따라서 Figs. 8과 9의 1:1 선에서 벗어나는 정도에 따라서 빙정의 크기 관측이 과대 크기(oversize) 또는 과소 크기(undersize) 됨을 알 수 있다. 이해를 돕기 위해 매 50% 과대 크기를 노란색 선으로, 매 20% 과소 크기를 옅은 파란색 선으로 표시했다. 또한 주어진 육각형 빙정의 크기(수평 축의 한 점)에 대해 전방산란 관측 기기가 복수의 구름입자 크기 산출이 가능한 경우 최대값과 최소값을 붉은색 점으로 표시하였다. 따라서 짙은 파란색 점은 복수의 산출된 구름입자 크기의 평균값이다. 산출된 구름입자의 크기의 과대 또는 과소 경향은 미 산란에서 간섭과 공명 효과에 때문에 단순하지 않고 매우 복잡하다. 뚜렷한 특징은 종횡비가 4.0인 장형(prolate)의 육각형 빙정의 경우 산출된 크기는 모두 과소 산출된다는 것이다. 이것은 전방산란(Fig. 8) 및 후방산란(Fig. 9)에서 모두 확인된다. 또한 전체적으로 편형(oblate, 종횡비 = 0.1) 또는 장형(종횡비 = 4.0) 모양의 육각형 빙정과 비교하면 육각형 빙정의 모양이 구형에 가까울수록(즉, 종횡비~1.0) 산출된 크기의 오차가 줄어듦을 알 수 있다.

Fig. 8.

Comparisons (blue circles) between actual sizes of hexagonal ice crystals (horizontal) and those determined (vertical) based on the scattering cross sections of hexagonal ice crystals and Mie calculations (i.e., current method used in forward scattering probes) in forward directions (4~12o) for AR indicated in panel. When determination of multiple sizes is possible a blue circle represents average value, while red circles are maximum and minimum of possible multiple sizes. The 1:1 line is shown with black broken line and every +50% (orange) and -20% (sky blue) from the 1:1 line are shown with in each panel.

Fig. 9.

Same as Fig. 8, but for backward directions (168~176o).

Figures 10과 11은 Figs. 8과 9의 계산을 종합하여 비구형인 빙정을 현재 항공관측에서 사용하고 있는 전방산란 관측 기기로 관측 하였을 때 구름입자 크기 결정의 오차를 전방산란(Fig. 10)과 후방산란(Fig. 11)에 대해, 또한 실제 구름 속에 존재하는 육각형 빙정의 변화 가능한 종횡비를 고려하여 정량화하였다. 구름입자 크기 결정의 오차(difference in diameter)는 100% × (Mie D_max - D_max)/D_max로 계산하였다. 여기서 D_max는 실제 육각형 빙정의 크기이고(Table 2), Mie D_max는 미 이론으로 계산한 실제 전방산란 관측 기기가 산출하는 크기이다. 따라서 양의 %는 전방산란 관측 기기가 빙정의 크기를 과대 산출한 것이고, 음의 %는 과소 산출을 의미한다. 구름입자의 크기 및 모양(즉, 종횡비) 변화에 따라서 전방산란 관측 기기의 빙정 크기 산출 오차가 매우 복잡하게 변한다. 전방산란 관측 기기의 관측 범위 전체에 걸친(즉, 2~50 μm) 크기 결정 오차의 절대값의 평균을 살펴보면, 전방산란의 경우(4~12^o, Fig. 10)는 종횡비 0.1, 0.25, 0.5, 1.0, 2.0, 4.0에 따라서 25.0 ± 13.1%, 19.3 ± 11.2%, 13.8 ± 10.9%, 18.8 ± 17.0%, 23.7 ± 12.4%, 39.6 ± 13.7%이며, 후방산란의 경우(168~176^o, Fig. 11)는 61.7 ± 15.0%, 42.1 ± 16.2%, 26.6 ± 15.5%, 44.5 ± 31.5%, 29.9 ± 21.4%, 58.8 ± 16.1%에 달한다. 전방산란의 경우 종횡비와 관계없이 최소 ~13% 이상, 후방산란의 경우 최소 ~26% 이상의 큰 오차를 보이고 있다. 하지만 이는 평균값이고 주어진 빙정 크기에 대해 복수의 크기 산출이 가능한 경우 이 오차는 더욱 증가한다. 예를 들어 종횡비 1.0인 육각형 빙정의 전방산란의 경우 특정 빙정 크기 산출의 오차는 최대 120%에 달하고, 후방산란의 경우 최대 132%에 달한다. 이는 매우 큰 오차이며 현재 전방산란 관측 기기의 관측에서 무시되고 있으나 전방산란 관측 기기를 사용한 빙정 관측의 정확도를 향상하기 위해서 반드시 개선되어야 하는 부분이다. 또한 전방산란과 후방산란에서 발생하는 구름입자 크기 결정의 오차를 비교해보면 후방산란의 경우가 더 크다. 예를 들어 종횡비 1.0인 육각형 빙정의 전방산란의 경우 평균 오차는 18.8 ± 17.0%인 반면 후방산란의 경우 44.5 ± 31.5%이다. 이는 후방산란 영역에 대해 빙정의 단일산란 특성(즉, 산란위상함수)이 빙정의 크기에 대해 더욱 민감함을 의미한다. 따라서, 전방산란과 동시에 후방산란을 함께 측정하는 CAS, CAS-POL, CPSPD (Table 1) 등의 관측 기기로 관측된 자료를 처리함에 각별한 주의가 요구된다. 전방산란과 후방산란을 함께 측정하는 관측 기기는 전방산란만을 관측하는 기기에 비해 더 많은 정보를 제공하지만, 자료 처리에 있어 애매성과 복잡성이 증가하는 단점이 있다.

Fig. 10.

Differences (100% × (Mie Dmax - Dmax)/Dmax) between actual sizes of hexagonal ice crystals and those determined based on spherical liquid droplets using Mie calculations in forward (4~12o) directions as a function of AR of hexagonal ice crystals (each panel). Red circles for a given size indicate maximum and minimum differences due to possible multiple sizes. Average and standard deviation of absolute values of blue circles are embedded in each panel.

Fig. 11.

Same as Fig. 10, but for backward directions (168~176o).

5. 요약 및 토의

항공기를 사용한 구름 관측에 사용하는 전방산란 관측 기기는 크기 50 μm 이하 구름입자의 수농도와 크기를 관측한다. 전방산란 관측 기기는 산란체(예, 구름입자)의 굴절률과 모양을 알고 있는 상태에서 특정 산란 각도(예, 전방산란 4~12^o 또는 후방산란 168~176^o)로 산란된 빛의 강도를 측정하면 산란체의 크기를 결정할 수 있다는 이론에 기반하여 개발되었다. 현재 사용하고 있는 이론은 미 이론으로서 구형의 액체상 구름입자를 가정하고 있기 때문에 비구형 고체상 물로 이루어진 빙정 관측 시 크기 결정의 오차가 발생한다. 이는 빙정이 고체상이기 때문에 발생하는 액체상과의 굴절률의 차이와 비구형 모양이 야기하는 특정 산란 각도로 산란된 빛의 강도의 차이 때문이다. 미 애매성과 비구형 빙정이 야기하는 크기 결정의 오차 문제 이외에도 전방산란 관측 기기는 여러 개의 구름입자들이 동시에 관측되어 하나의 큰 구름입자 크기로 산출되고 수농도는 낮게 관측되는 문제(coincidence problem), 액체상의 구름입자나 고체상의 빙정이 관측 기기의 돌출된 부위에 부딪혀 여러 개의 작은 크기로 부서져서 크기는 작게 산출되고 수농도는 높게 관측되는 splashing과 shattering 등의 문제를 갖고 있다. 항공기를 사용한 구름 관측에서 사용되는 관측 기기의 작동 원리, 문제점, 보정 방법 등에 관한 연구는 지속적으로 진행 중이다(Baumgardner et al., 2012, 2017; Wendisch and Brenguier, 2013; McFarquhar et al., 2017).

비구형 빙정이 야기하는 전방산란 관측 기기 관측 오차를 정량화하기 위해 선행연구(Borrmann et al., 2000; Meyer, 2012)는 회전대칭 모양인 원기둥과 회전타원체를 빙정 모양으로 가정하고 T-matrix 방법을 사용한 계산을 통해 크기 결정에 약 20%의 오차가 존재함을 보고했다. 하지만 실제 작은 빙정은 빙정의 분자들이 육각 격자 구조를 갖기 때문에 기본적으로 육각형 모양이다. 따라서 본 연구에서는 육각형 모양을 갖는 빙정의 단일산란 특성(즉, 산란위상함수와 산란 단면)을 ADDA 방법을 사용하여 계산했다. 또한 육각형 빙정의 자연적인 모양 변화 범위를 고려하기 위해 여섯 개의 다른 종횡비를 갖는 육각형 빙정 모형을 사용했다.

전방산란 관측 기기의 관측 범위에 대해 계산한 비구형 육각형 빙정의 산란위상함수(Fig. 6)와 미분산란 단면(Fig. 7)은 구형의 구름입자 계산과는 큰 차이를 보였다. 산출된 구름입자의 크기 결정의 과대 또는 과소 경향은 미 산란에서 발생하는 간섭과 공명 효과 때문에 매우 복잡하며, 편형(종횡비 = 0.1) 또는 장형(종횡비 = 4.0) 모양의 육각형 빙정과 비교하면 육각형 빙정의 모양이 구형에 가까울수록(즉, 종횡비~1.0) 산출된 크기의 오차가 줄어듦을 알 수 있다(Figs. 8, 9). 전방산란 관측 기기로 비구형 빙정을 측정 시 발생하는 크기 결정의 오차는 전방산란의 경우 종횡비와 관계없이 평균 최소 ~13% 이상(Fig. 10), 후방산란의 경우 평균 최소 ~26% 이상이다(Fig. 11). 주어진 빙정 크기에 대해 복수의 크기 결정이 가능한 경우 이 오차는 전방산란의 경우 최대 120%, 후방산란의 경우 최대 132%에 달한다. 이 결과는 회전체를 가정하여 계산한 선행연구의 결과(약 20%)와 비교 시 매우 크며, 전방산란 관측 기기의 정확도 향상을 위해서 후처리 과정에서 반드시 고려해야 한다.

McFarquhar et al. (2007)은 50 μm보다 작은 구름입자 크기 분포가 위성 원격탐사에서 사용하고 있는 구름 특성 산출 방법과 수치 모형에서 구름의 표현에 중요하다는 것을 강조했다. 또한 전방산란 관측 기기를 사용하는 항공기 관측의 어려움과 개선에 대하여 설명하였다. 본 연구에서는 전방산란 관측 기기를 사용하여 비구형 빙정의 크기 관측 시 발생하는 오차를 정량화 하였다. 이 오차는 무시할 수 없으며 구름입자 크기 분포 측정의 정확도 향상을 위해서 후처리 시 반드시 고려해야 한다. 2017년 12월 대한민국 기상청은 연구용 항공기를 도입하였다(Cha et al., 2019). 이 항공기에는 CDP라는 전방산란 관측 기기가 장착되어 관측을 수행 중이다. 구름물리, 원격탐사, 수치모형 연구 분야의 발전을 위해서는 더욱 정확한 구름 관측이 필요하며, 본 연구 결과는 구름입자 관측, 특히 작은 빙정 크기 관측 정확도 향상에 기여할 것이다. 향후 본 연구를 더욱 발전시켜 기상청 CDP 관측 자료 후처리에 활용할 계획이다.

Acknowledgments

이 논문은 부산대학교 기본연구지원사업(2년)에 의하여 연구되었음. 본 논문의 개선을 위해 좋은 의견 제시해 주신 두 분의 심사위원님께 감사드립니다.

References

• Bailey, M., and J. Hallett, 2004: Growth rates and habits of ice crystals between -20^o and -70^oC. J. Atmos. Sci., 61, 514-544. [https://doi.org/10.1175/1520-0469(2004)061<0514:GRAHOI>2.0.CO;2]
• Baumgardner, D., H. Jonsson, W. Dawson, D. O’Connor, and R. Newton, 2001: The cloud, aerosol and precipitation spectrometer: a new instrument for cloud investigations. Atmos. Res., 59-60, 251-264. [https://doi.org/10.1016/S0169-8095(01)00119-3]
• Baumgardner, D., H. Chepfer, G. B. Raga, and G. L. Kok, 2005: The shapes of very small cirrus particles derived from in situ measurements. Geophys. Res. Lett., 32, L01806. [https://doi.org/10.1029/2004GL021300]
• Baumgardner, D., and Coauthors, 2012: In situ, airborne instrumentation: Addressing and solving measurement problems in ice clouds. Bull. Amer. Meteor. Soc., 93, 29-34. [https://doi.org/10.1175/BAMS-D-11-00123.1]
• Baumgardner, D., R. Newton, M. Krämer, J. Meyer, A. Beyer, M. Wendisch, and P. Vochezer, 2014: The Cloud Particle Spectrometer with Polarization Detection (CPSPD): A next generation open- path cloud probe for distinguishing liquid cloud droplets from ice crystals. Atmos. Res., 142, 2-14. [https://doi.org/10.1016/j.atmosres.2013.12.010]
• Baumgardner, D., and Coauthors, 2017: Cloud ice properties: In situ measurement challenges. Meteor. Monogr., 58, 9.1-9.23. [https://doi.org/10.1175/AMSMONOGRAPHS-D-16-0011.1]
• Bi, L., and P. Yang, 2014: Accurate simulation of the optical properties of atmospheric ice crystals with the invariant imbedding T-matrix method. J. Quant. Spectrosc. Radiat. Transfer, 138, 17-35. [https://doi.org/10.1016/j.jqsrt.2014.01.013]
• Borrmann, S., L. Beiping, and M. Mishchenko, 2000: Application of the T-matrix method to the measurement of aspherical (ellipsoidal) particles with forward scattering optical particle counters. J. Aerosol Sci., 31, 789-799. [https://doi.org/10.1016/S0021-8502(99)00563-7]
• Cha, J. W., and Coauthors, 2019: Analysis of results and techniques about precipitation enhancement by aircraft seeding in Korea. Atmosphere, 29, 481-499 (in Korean with English abstract). [https://doi.org/10.14191/Atmos.2019.29.4.481]
• Cox, C. J., D. C. Noone, M. Berkelhammer, M. D. Shupe, W. D. Neff, N. B. Miller, V. P. Walden, and K. Steffen, 2019: Supercooled liquid fogs over the central Greenland Ice Sheet. Atmos. Chem. Phys., 19, 7467-7485. [https://doi.org/10.5194/acp-19-7467-2019]
• Dye, J. E., and D. Baumgardner, 1984: Evaluation of the forward scattering spectrometer probe. Part I: Electronic and optical studies. J. Atmos. Ocean. Tech., 1, 329-344. [https://doi.org/10.1175/1520-0426(1984)001<0329:EOTFSS>2.0.CO;2]
• Glen, A., and S. D. Brooks, 2013: A new method for measuring optical scattering properties of atmospherically relevant dusts using the Cloud and Aerosol Spectrometer with Polarization (CASPOL). Atmos. Chem. Phys., 13, 1345-1356. [https://doi.org/10.5194/acp-13-1345-2013]
• Gonser, S. G., O. Klemm, F. Griessbaum, S.-C. Chang, H.-S. Chu, and Y.-J. Hsia, 2012: The relation between humidity and liquid water content in fog: An experimental approach. Pure Appl. Geophys., 169, 821-833. [https://doi.org/10.1007/s00024-011-0270-x]
• Knollenberg, R. G., 1970: The optical array: An alternative to scattering or extinction for airborne particle size determination. J. Appl. Meteor., 9, 86-103. [https://doi.org/10.1175/1520-0450(1970)009<0086:TOAAAT>2.0.CO;2]
• Knollenberg, R. G., 1976: Three new instruments for cloud physics measurements: The 2-D spectrometer probe, the forward scattering spectrometer probe, and the active scattering aerosol spectrometer. Preprints, Int. Conf. on Cloud Physics, Amer. Meteor. Soc., 554-561.
• Knollenberg, R. G., 1981: Techniques for probing cloud microstructure. In P. V. Hobbs and A. Deepak, Eds., Clouds - Their Formation, Optical Properties and Effects, Academic Press, 15-91. [https://doi.org/10.1016/B978-0-12-350720-4.50007-7]
• Lance, S., C. A. Brock, D. Rogers, and J. A. Gordon, 2010: Water droplet calibration of the cloud droplet probe (CDP) and in-flight performance in liquid, ice and mixed-phase clouds during ARCPAC. Atmos. Meas. Tech., 3, 1683-1706. [https://doi.org/10.5194/amt-3-1683-2010]
• Lawson, R. P., R. E. Stewart, and L. J. Angus, 1998: Observations and numerical simulations of the origin and development of very large snowflakes. J. Atmos. Sci., 55, 3209-3229. [https://doi.org/10.1175/1520-0469(1998)055<3209:OANSOT>2.0.CO;2]
• Lawson, R. P., D. O’Connor, P. Zmarzly, K. Weaver, B. Baker, Q. Mo, and H. Jonsson, 2006: The 2DS (stereo) probe: Design and preliminary tests of a new airborne, high-speed, high-resolution particle imaging probe. J. Atmos. Oceanic Technol., 23, 1462-1471. [https://doi.org/10.1175/JTECH1927.1]
• Lawson, R. P., and Coauthors, 2019: A review of ice particle shapes in cirrus formed in situ and in anvils. J. Geophys. Res., 124, 10049-10090. [https://doi.org/10.1029/2018JD030122]
• Macke, A., J. Mueller, and E. Raschke, 1996: Single scattering properties of atmospheric ice crystals. J. Atmos. Sci., 53, 2813-2825. [https://doi.org/10.1175/1520-0469(1996)053<2813:SSPOAI>2.0.CO;2]
• McFarquhar, G. M., J. Um, M. Freer, D. Baumgardner, G. L. Kok, and G. Mace, 2007: Importance of small ice crystals to cirrus properties: Observations from the Tropical Warm Pool International Cloud Experiment (TWP-ICE). Geophys. Res. Lett., 34, L13803. [https://doi.org/10.1029/2007GL029865]
• McFarquhar, G. M., and Coauthors, 2017: Processing of Ice Cloud In Situ Data Collected by Bulk Water, Scattering, and Imaging Probes: Fundamentals, Uncertainties, and Efforts toward Consistency. Meteor. Monogr., 58, 11.1-11.33. [https://doi.org/10.1175/AMSMONOGRAPHS-D-16-0007.1]
• Meyer, J., 2012: Ice Crystal Measurements with the New Particle Spectrometer NIXE-CAPS. Forschungszentrum Jülich GmbH, Institute for Energy and Climate Research, 132 pp.
• Mishchenko, M. I., and L. D. Travis, 1998: Capabilities and limitations of a current Fortran implementation of the T-matrix method for randomly oriented, rotationally symmetric scatterers. J. Quant. Spectrosc. Radiat. Transfer, 60, 309-324. [https://doi.org/10.1016/S0022-4073(98)00008-9]
• Mishchenko, M. I., L. D. Travis, and D. W. Mackowski, 1996: T-matrix computations of light scattering by nonspherical particles: A review. J. Quant. Spectrosc. Radiat. Transfer, 55, 535-575. [https://doi.org/10.1016/0022-4073(96)00002-7]
• Pinnick, R. G., and H. J. Auvermann, 1979: Response characteristics of Knollenberg light-scattering aerosol counters. J. Aerosol Sci., 10, 55-74. [https://doi.org/10.1016/0021-8502(79)90136-8]
• Pinnick, R. G., D. M. Garvey, and L. D. Duncan, 1981: Calibration of Knollenberg FSSP light-scattering counters for measurement of cloud droplets. J. Appl. Meteor. Climatol., 20, 1049-1057. [https://doi.org/10.1175/1520-0450(1981)020<1049:COKFLS>2.0.CO;2]
• Sassen, K., Z. Wang, and D. Liu, 2008: Global distribution of cirrus clouds from CloudSat/Cloud-Aerosol Lidar and Infrared Pathfinder Satellite Observations (CALIPSO) measurements. J. Geophys. Res., 113, D00A12. [https://doi.org/10.1029/2008JD009972]
• Schnaiter, M., S. Büttner, O. Möhler, J. Skrotzki, M. Vragel, and R. Wagner, 2012: Influence of particle size and shape on the backscattering linear depolarisation ratio of small ice crystals - Cloud chamber measurements in the context of contrail and cirrus microphysics. Atmos. Chem. Phys., 12, 10465-10484. [https://doi.org/10.5194/acp-12-10465-2012]
• Spiegel, J. K., P. Zieger, N. Bukowiecki, E. Hammer, E. Weingartner, and W. Eugster, 2012: Evaluating the capabilities and uncertainties of droplet measurements for the fog droplet spectrometer (FM-100). Atmos. Meas. Tech., 5, 2237-2260. [https://doi.org/10.5194/amt-5-2237-2012]
• Um, J., and G. M. McFarquhar, 2007: Single-scattering properties of aggregates of bullet rosettes in cirrus. J. Appl. Meteor. Climatol., 46, 757-775. [https://doi.org/10.1175/JAM2501.1]
• Um, J., and G. M. McFarquhar, 2009: Single-scattering properties of aggregates plates, Q. J. Roy. Meteor. Soc., 135, 291-304. [https://doi.org/10.1002/qj.378]
• Um, J., and G. M. McFarquhar, 2011: Dependence of the single-scattering properties of small ice crystals on idealized shape models, Atmos. Chem. Phys., 11, 3159-3171. [https://doi.org/10.5194/acp-11-3159-2011]
• Um, J., and G. M. McFarquhar, 2013: Optimal numerical methods for determining the orientation averages of single-scattering properties of atmospheric ice crystals. J. Quant. Spectrosc. Radiat. Transfer, 127, 207-223. [https://doi.org/10.1016/j.jqsrt.2013.05.020]
• Um, J., and G. M. McFarquhar, 2015: Formation of atmospheric halos and applicability of geometric optics for calculating single-scattering properties of hexagonal ice crystals: Impacts of aspect ratio and ice crystal size. J. Quant. Spectrosc. Radiat. Transfer, 165, 134-152. [https://doi.org/10.1016/j.jqsrt.2015.07.001]
• Um, J., G. M. McFarquhar, Y. P. Hong, S.-S. Lee, C. H. Jung, R. P. Lawson, and Q. Mo, 2015: Dimensions and aspect ratios of natural ice crystals. Atmos. Chem. Phys., 15, 3933-3956. [https://doi.org/10.5194/acp-15-3933-2015]
• Wendisch, M., and J.-L. Brenguier, 2013: Airborne Measurements for Environmental Research: Methods and Instruments. Wiley and Sons, 641 pp. [https://doi.org/10.1002/9783527653218]
• Yurkin, M. A., and A. G. Hoekstra, 2007: The discrete dipole approximation: an overview and recent developments. J. Quant. Spectrosc. Radiat. Transfer, 106, 558-589. [https://doi.org/10.1016/j.jqsrt.2007.01.034]
• Yurkin, M. A., and A. G. Hoekstra, 2011: The discrete-dipole-approximation code ADDA: capabilities and known limitations. J. Quant. Spectrosc. Radiat. Transfer, 112, 2234-2247. [https://doi.org/10.1016/j.jqsrt.2011.01.031]